Introduction
It is a platitude, denied by very few, that minds causally interact with the
world. Causal theories of mental content hold that mental states represent
the world in virtue of the sorts of causal relationships they have within the
mind and with the world. Not all theories of mental representation are
causal in nature. For example, Locke's notion of the ideas of primary
qualities was that they represented qualities in an object, not through reliable
causation, but because they were similar (share the same properties.). Contemporary causal theories of mental content have predecessors dating
back to Book III of John Locke's Essay Concerning Human Understanding, possibly even Aristotle's
De Anima, or even to Plato's Theatetus. Locke's notion of
secondary qualities (a quality or power of the object to cause particular ideas
in us which bear no similarity to the object, e.x. color) looks very much like a
contemporary causal theory called nomic covariation. (Cummins 1989). Aristotle's
discussion in Book II of De Anima suggests that, for example, color in
the object is different from our sensations of color, which are nevertheless
reliably caused by light hitting the object. Similarly, Plato's analogy of
perception as the matching of sensations caused by the world to impressions
(knowledge) within the wax also suggests that Plato entertained the notion that
causal connections allowed our minds to represent. Causal theories develop in the context of some or other
representational theory of mind (RTM). A representational theory of mind
holds that mental states have intentionality (are about the world) in virtue of
a representational relationship holding between the mental state and the
object. Contemporary causal theories
of mental representation have developed in the theoretical on context
of explanation in Cognitive Science. To better understand these theories
it is helpful to view their intended role as an explanatory primitive in
computation explanations in Cognitive Science.
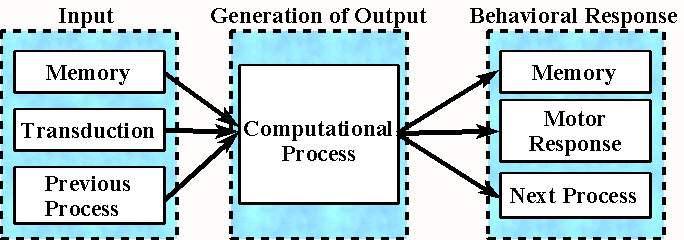
To cognize is to know, and to have a cognitive capacity is to have a capacity (ability) which seems to generate or require knowledge. Cognitive Science seeks to explain the cognitive capacities, of humans, animals, and possibly machines. However, the primary thrust of research in Cognitive Science is directed towards explaining human cognitive abilities. More recently, theorists have devoted attention to consciousness and emotion. The classic approach to explaining cognitive capacities within cognitive science has two foundational theses; The "Computational Theory of Cognition" (CTC) and the "Representational Theory of Intentionality" (RTI). Together these theses dictate the explanatory structure off Cognitive Science. The CTC characterizes cognition as the computation of complex functions on representational states, where computation consists in performing operations defined over representational states. These operations can be rule-based manipulations of syntactic strings as in traditional artificial intelligence, or the weighted passing of activation as in connectionist models. The RTI asserts that mental states have intentionality (are about the world/have content) in virtue of a representation relation holding between the world and those states. There are three conceptually distinct (though not necessarily temporally distinct) stages. CTC/RTI portrays cognizers as receiving input through sensory organs and/or memory and generating outputs in the form of memories, inputs to other processes, and/or motor response commands.

Cognitive Scientists hotly debate the exact nature of the computational
processes involved in cognition. On the one hand, many theorists
favor Turing-compatible computation and its development in contemporary
computer science and mathematics. On the other hand, many theorists favor
dynamically described state transitions (ex. Connectionism).
A similar theoretical dichotomy fosters debate in the literature on representation.
The two general theoretical approaches towards a theory of representation
are "
Nomic (law-like) Covariation" and "Functional Role Semantics".
Neither theoretical approach regarding the representation relation has gained general
acceptance as the theory presupposed
by the RTI. However, there are several
agreed-upon constraints for a theory of representation.
First, the representation relation must be consistent with the physicalistic
nature of Cognitive Science. Second, the relation
must be present and explanatory in accepted explanations within Cognitive
Science. (an appropriate characterization of "accepted" explanations is
somewhat controversial). Philosophers particularly would also like
to see the accepted theory of representation explain many of the widely
accepted properties of belief. Block (1986) and Fodor (1988) provide
excellent discussions of the potential problems, the solutions to which
many Philosophers hope to find in a theory of mental representation.
Theories
One theoretic approach to articulating the representation relation,
Nomic Covariation, postulates a simple causal relationship between the
object or property and the state that represents the object or property.
The other approach, Functional Role Semantics, hypothesizes that a state
has content in virtue of the state's occupying a particular position in
a complex web of causal relationships characterizing the cognizer's functioning. Candidate content-fixing causal relationships
include causal relationships within the cognizer (i.e., relations between
brain states) and/or without (i.e., relations to the distal environment).
Both Functional Role Semantics and Nomic Covariation theories satisfy the (above)
first,
physicalistic constraint by hypothesizing that a cognizer's states represent
the distal environment solely as a result of the specific sorts of causal
connections had by those states. The theories diverge in terms of
the specific causal relationships each emphasizes.
Nomic Covariation
Nomic covariation theories hypothesize a causal connection between
an object or property in the world and the state that represents that object
or property within the cognizer. Information theory as well
as work within a sub-field of psychology called psychophysics inspire contemporary
Nomic Covariance theorists. Fodor, for instance, notes that
...there are circumstances such that red instantiations control 'red' tokenings whenever those circumstances obtain; and it's plausible that 'red' expresses the property red in virtue of the fact that red instantiations cause 'red' tokenings in those circumstances; and the circumstances are nonsemantically, nonteleologically, and nonintentionally specifiable.
In fact, they're psychophysically specifiable. (Fodor 1988, p.112)
Covariation theories, as a result, seem to satisfy the second constraint upon theories of representation, i.e., that the relation must be present and explanatory in accepted explanations within Cognitive Science. For example, Hubel and Wiesel (1977) investigate the representational content of cells in the striate (visual) cortex by monitoring the activity of those cells looking for nomic preferential relationships between the activity of these cells and the presence of properties in the visual field. A system's states represent those objects and/or properties of the distal world with which they covary according to Nomic Covariation theories. Specifically, Nomic covariation theories assign contents to states via some version of the following definition (Cummins 1989, Fodor 1988):
State, Sc, represents C iff the system tokens Sc when, only when, and because of C.
Illustrative
animation
The intuitive appeal of Nomic Covariation stems from the idea that
a cognizer represents an object or property by being causally "in tune"
with that object or property. Further, Nomic Covariationists such
as Jerold Fodor advocate this approach because it assigns content to individual
states, Sc independent of how the cognizer operates upon
those states (inferences it can make) and/or the content of other states
with which Sc might interact. Fodor refers to this
assignment of content as "Punctate Content, or "atomic content."
Among the advantages claimed by Fodor for punctate content is that it allows
for content identity across individuals who have quite different theories
regarding some object or property. Similarly, punctate content provides
the only theory whereby people can refer to real objects even when they
have great numbers of false beliefs about them. For instance, Fodor
claims (1992) that Aristotle thought about and talked about the same things
we refer to by "stars" even though he falsely believed stars to be relatively
close and rotating around the Earth on glassy spheres. A final perceived
advantage for Nomic Covariation approaches lies in that they provide a
clear-cut mechanism through which a cognizer can come to know about an
object or property in the world in virtue of its representational capacities.
A cognizer that represents an object or property in the environment does
so insofar as the cognizer can reliably detect the object's presence.
Problems for Nomic Covariation
Semantic Reduction
Within Philosophy particularly, but throughout Cognitive Science, theorists
raise three standard objections to Nomic Covariation theories. The first
objection challenges the ability of covariation theories to explain the
representational capacities of states with regard to objects/properties
beyond low-level perceptual properties (e.x. color), especially very abstract,
high-level objects and properties like justice or electron.
Nomic covariationists suppose that law-like covariation determines the
content of states representing properties ranging from low-level properties
like red or zero-crossing , to ordinary, medium sized objects
and properties like table or cow. States represent properties
and objects of a higher level of abstraction, like electron, in
virtue of their being defined in terms of the representational properties
of lower level states. (Fodor 1988)
Robert Cummins (1989) objects to this strategy, claiming that the covariation of mental states with objects/properties requires that the cognizer represent heuristic information either implicitly or explicitly. Thus, nomic covariation will not explain the representational properties of any states but the most primitive representational states. All higher level concepts from dog to democracy must get meaning through definition. At first glance, it seems plausible to suppose that states that represent high-level theoretical properties like electron get their meaning through definition in terms of lower level properties. After all, such properties and objects often have theoretical definitions. However, the failure of such semantic reductions has shaped the history of Western Philosophy and Mathematics (Quine 1951). Worse still, the lower the level of abstraction, the more ordinary the concept, the less readily one accepts that one knows its meaning because one has an explicit definition for it. For example, covariation�s critics ask, if the state one uses to represent crow gets its meaning from being defined in terms of lower level properties, then why does one have difficulty in articulating that definition in any but the most superficial sense? Psychological research also appears to run contrary to semantic reductionism in that it indicates that object recognition and categorization are strongly influenced by perceptual features, the exact make-up of which is somewhat fluid.
According to Cummins, mental states achieve covariation with cats because
we already have explicitly represented or implicit knowledge about cats
(ex. they are domesticated felines). Further, if covariation
is not the simple, unmediated causal relationship between the property/object
and the state, but rather the result of often complex causal interactions
within the system, then this suggests that Nomic Covariation is really just another
instance of its competitor, Functional Role Semantics.
The Disjunction Problem
The second, and most widely discussed problem for Nomic Covariation
theories is called the "Disjunction Problem". According to covariationists,
a state represents an object or property if the system tokens (enters into)
that state when confronted with the object or property and ONLY WHEN
confronted with that property. However, suppose that cognizer tokens a
state, S c, in all of it�s cat interactions. According
to the WHEN clause of the covariationsist definition, the cognizer�s
state appears to represent cathood. Unfortunately, one night the cognizer
tokens an S c when seeing a skunk in the darkness. The
ONLY
WHEN clause of the definition prima facie dictates that
Sc
never really represented cathood simpliciter. Rather, the cognizer has always represented
a property that we can only describe using the disjunction, "cat or skunk".
Illustrative
Animation
The disjunction problem poses difficulties for Nomic Covariation theories in two ways: First, the theory seems to dictate counterintuitive representational contents for states. If one has beliefs about cats, (e.x. cats are domesticated felines that have a number of distinct breeds), then Nomic Covariation seems to dictate that those beliefs have always been about things having the property of being either a cat or a skunk. Second, the disjunction problem seems to demonstrate that Nomic Covariation cannot account for misrepresentation, since any seeming case of misrepresentation by a cognizer becomes a correct representation of a disjunctive property under Nomic Covariation. In other words, one never mistakenly believes that one sees one�s mother at the corner, one always correctly believes that "mother/other" is at the corner.
Theorists have explored a number of solutions to the disjunction problem. All of these solutions rely upon some form of the idealization strategy: A given solution will separate cases of the tokening (occurrence) of a state into two groups, one in which content is already fixed and representational error can occur (normal conditions), and the second class (ideal circumstances) in which content is fixed by perfect covariation. There are several important versions of this solution:
Ideal Conditions
Advocates of the ideal conditions solution to the disjunction problem
suggest one represents "mother" and not "mother/other" despite occasionally
mistaking others for mothers because under ideal perceptual conditions
(i.e., in good light, at close distance, etc.) one can distinguish mothers
from others. Idealization in mainstream science is both the inspiration
behind the covariationist's idealization strategy and its source of tacit
plausibility. In normal cases of scientific idealization theorists ignore certain
negligible parameters in real systems to formulate a law. The resulting
law, though not strictly true of any actual system, proves predictively
adequate, and quantifies a real relation in actual physical systems.
For example, real gases are not composed of Newtonian molecules in that
they are not point masses, nor are their collisions perfectly elastic.
Likewise, heat is not the only energy source present in gases. In most
cases, however, other parameters (like electromagnetic forces) prove negligible.
One idealizes away from such negligible parameters to quantify the inverse
relationship between, on the one side, the pressure and volume of a gas,
and on the other side, the temperature and number of moles. Idealizing
away from other parameters results in the ideal gas law: PV = nRT.
The move toward idealization in response to the disjunction problem attempts to emulate noncontroversial cases of scientific idealization like the above-described ideal gas law. The basic idealization move defines representation as follows:
A state Sc represents Cs as Cs iff under ideal circumstances the system tokens a Sc when, only when, and because Cs are present.
On the idealization line, Sc represents cats as cats since, under ideal circumstances, the cognizer tokens Scs when, only when, and because instances of cathood are present. State Sc does not represent "cat or skunk" because under ideal circumstances skunks do not cause the cognizer to token Sc. Such an idealization from error, admits the covariationist, breaks down in abnormal circumstances. Nevertheless, the idealization allows psychological laws to capture a real relation (the representation relation) in actual systems. Moreover, since most cases are close to ideal circumstances, psychological laws utilizing idealized nomic covariation prove predictively adequate.
The nomic covariationist's ideal conditions solution divides tokenings of a state by a system into content-imbuing (ideal) and content-fixed (malfunctioning/atypical) classes. Covariationists assert that one legitimately idealizes away from error because errors are coextensive with the cases of malfunction and/or atypical situations from which one legitimately idealizes. For example, if one pulls a weighted spring hard enough to bend or break the spring, it will no longer obey the standard harmonic oscillation equation. Such spring systems do not undermine the the basic physics of dampened harmonic oscilatory systems because the circumstances are such that the spring system cannot operate qua spring system. The same holds for cognitive malfunctions: One's tokening of a Sc as a result of a amphetamine induced psychosis does not introduce disjunctive content because amphetamine induced psychosis is a case of mental malfunction.
Of course, all skunk-caused instances of Sc will
not trace their origins to malfunction. One could token a Sc
as a result of the same cat-like features prompting tokenings of Sc
for cats. Dismissing such look-alike cases as malfunctioning requires
one also dismiss cat cases as malfunctions. All cognition becomes
malfunction, if one focuses exclusively upon malfunction in specifying
ideal conditions. Consider another example.
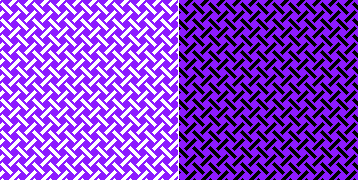
Illustrative
Animation
Subjects normally judge the right side of the above figure to have
a much darker shade of purple than the left side. In fact, the surface
reflectance of the purple areas of the figure remains uniform (it is all
the same color of purple). Psychologists refer to the mistaken judgment as "the assimilation effect". Psychologists explain the assimilation
effect by attributing the mistake to the pooling together of signals from
several retinal cells. In most circumstances, pooling reduces equivocation
(error or noise) that is due to false signals from individual retinal cells.
However, when one closely intersperses the dark and light patterns throughout
a visual field, pooling serves to inhibit or to excite cell firing, resulting
in a misrepresentation of relative shading. So, a case of normal
functioning--in fact, functioning that acts to reduce error--results in
misrepresentation.
Nomic Covariance theorists respond to such normal functioning cases by appealing to atypical conditions. For instance, when the pressure upon a gas exceeds atmospheric level (1.013 x 105 N/m2), or when its temperature becomes too great, the negligible parameters ignored by the idealized gas law become non-negligible. The predictive accuracy of the idealized gas law plummets, though the gas does not malfunction. Nevertheless, these cases do not count against the idealized gas law because the circumstances are atypical. The nomic covariationist likewise claims that circumstances are perceptually atypical, that is, less than ideal, when the system tokens a Sc in response to a cat.
The covariationists' move looks suspiciously circular unless they can
specify an independent means of ruling out case of similar features as atypical.
In other words, one must define ideal conditions so that malfunction and
atypical circumstances prove coextensive with error. But one cannot
avail oneself of the notion of error, nor of other intentional or semantic
notions in formulating and motivating the definition. One must have
a reason for labeling the skunk to Sc cases as less than ideal, and the reason
cannot be that skunks cause cat tokens (Sc) in feature
similarity cases. For example, in the movie "The Crying Game" an
Irish man trying to escape from the IRA becomes romantically involved with
a woman. However, much to the surprise of the Irish man (and the
audience) this woman is actually a man. The reaction of the
Irish man clearly shows he misrepresented the gender of his romantic partner.
Yet, the Irish man did see his partner in good light, at close distance,
etc.. The natural solution to this difficulty attempts to take advantage
of the idea that there are conditions under which such a sex difference
would not escape notice. However, in specifying such conditions as
ideal conditions for this case, one must be guided in a circular manner
by one's knowledge of the property that the state actually represents.
Additionally, critics (Wallis 1994 and 1994a) argue that appealing to idealization to defeat the disjunction problem
is strongly and negatively disanalogous with successful uses of idealization
is science. For example, in order to explain misrepresentation in cases of
malfunction or atypical circumstances, the covariationist must rely upon the
content dictated by idealized nomic covariation. When a skunk causes
an Sc because bad lighting, the nomic covariationist must
suppose that Sc represents cats in order to explain why the
person misrepresented the skunk as a cat. Thus, for the covariationist the
results of the idealization are applicable in conditions which violate the
presuppositions of the idealization. In contrast, when the pressure
upon a gas exceeds atmospheric level (1.013 x 105 N/m2), or when its temperature
becomes too great, the negligible parameters ignored by the idealized gas
law become non-negligible. In these atypical circumstances physicists will
agree that the ideal gas law does not apply since the presuppositions of the
idealization do not hold. Thus, while the mainstream use of idealization
is limited to ideal or close to ideal circumstances, the covariationist
cannot accept such limitations.
Learning Periods
Fred Dretske (1981, 1989) has suggested that content
gets fixed for a state during a learning period. In this period,
cognizer develops a perfect causal connection between the state and the
object or property with the help of an instructor who provides examples
and corrections. Once the learning period ends, the cognizer's state
has a fixed content and tokenings of the state in cases where the object
or property is not present count as misrepresentations.
Fodor (1988) and others have criticized this approach
on two grounds. First, there seems to be no principled distinction
between learning and non-learning periods. Hence, there are no grounds for calling
some tokenings of the state content imbuing and others representing or
misrepresenting. Second, even if one could specify a learning period,
there seems to be no principled distinction between the univocal and disjunctive
causal connections. Critics ask why, if one tokens Sc
for both cat and skunk in the post-learning period, one shouldn't suppose that the
causal connection created in the learning period is between
cats and Sc and not between cat/skunk and Sc?
Teleological Accounts
Advocates of a teleological solution to the disjunction
problem, such as Ruth Millikan (1983 and 1986), suggest that evolutionary
history determines content. For example, a frog captures and eats
any ambient moving dot in its visual field. As a result, hungry frogs
eat ball-bearings rolled in front of them. Advocates of the teleological
account claim that frogs misrepresent ball-bearings as flies because the
function of a frog's visual cells is determined by the uses of those cells
responsible for a frog's ability to propagate it's genome, i.e., uses involving
fly capturing.
Fodor (1988) criticizes teleological accounts on
the ground that evolution does not select with sufficient precision to
account for typical univocal content claims. If a frog represents
ambient dots as fly or ball-bearing in a fly-rich, ball-bearing-poor environment,
then that disjunctive representational content would account for the frog's
ability to propagate its genome. Hence, claims Fodor, evolutionary history will
not favor fly over fly-or-ball-bearing as the content of frog visual cells.
Two other objections to teleological accounts appear
in the literature. First, most artifacts (e.x., compact discs, SUVs,
etc.) in the human environment were not present during a significant portion
of human evolutionary history. As a result, advocates of teleological
solutions to the disjunction problem must explain a huge percentage of
the representational capacities of humans, including the representations
of many ordinary objects like chairs or beer, by definition. Second, because teleological accounts appeal to the evolutionary
history of a cognizer to explain representational abilities, any seeming
representational abilities without such a history would not have representational
content on the teleological account
(Cummins 1989). For example, if a molecule for
molecule duplicate of Millikan where to spontaneously appear, it would
seem to have all of her cognitive/representational abilities.
Yet, on Millikan's account, her exact double would not have any states
with representational content. In other words, one cannot appeal
to the adaptational significance of a state to explain its representational
content, since in order for the state to have adaptational significance
it must play a part in the cognitive capacities the system, and hence already
have representational content.
Asymmetric Dependence
Fodor's (1990) asymmetric dependence theory suggests
another twist on the general idealization strategy. In addition to
a nomic connection between a state, Sc, and an object/property, cathood,
asymmetric dependence theories define representation in terms of two counterfactual
scenarios: If, skunks cause Scs, then (1) breaking the
skunk to Sc connection does not break the cat to Sc
and (2) breaking the
cat to Sc connection does break the skunk to Sc
connection. As above,
potentially troublesome cases get ruled out because there is a counterfactual
set of conditions in which subjects can distinguish cats from skunks.
Critics (Cummins, 1989 and Wallis 1995) argue that asymmetric dependence
theories fare no better than other versions of nomic covariation.
One difficulty is that the brain recognizes higher level concepts through
the detection of features. As a consequence, it appears that there
is no asymmetric dependence between cases, or worse, it goes the wrong
way from fake to representation. For instance, if I find my
car by looking for features x,y,z, then I can break the car to Scar connection
by altering the appearance of my car. But that will not break the look-alike
car to Scar connection (violating 2). If, on the other hand,
I break the look-alike car to Scar connection (say by altering my
beliefs about my car's appearance), then it seems I do break the
car to Scar connection (violating 1). Similar stories can be told
in terms of the normal, albeit somewhat noisy, functioning of cells in
the visual system. (Wallis 1995).
Failure of Univocal Feature Detection for Low-Level Properties
Finally, some theorists like Kathleen Akins (1996) (also Churchland and Sejnowski 1992 and Wallis 1995) argue that the various cells that perform sensory transduction are not properly characterized as the sorts of feature detectors required by nomic covariation. Such sensory cells do not respond exclusively to the presence of some particular object or property, but can and do respond to other properties. Their responses are not all or nothing. Rather, they show "selective sensitivity" to properties in that they respond to a wider range of stimulus, but respond more strongly to certain stimuli. For example, rods and cones in the retina are often portrayed as representing a certain wavelength of light. The response curves of these cells in fact overlap dramatically.
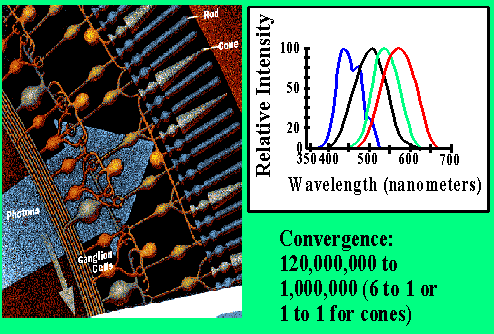
Thus,
the same response can be elicited from a red cone by similar levels of blue or
green light. Additionally, perception is riddled by often useful confabulations
by the sensory systems. For example, rod and cone activation is
represented by averages of pooled cells almost immediately in the visual
system. This has a good effect in that it eliminates noise from sources
like body heat, but can also lead to the assimilation
effect noted earlier. Similarly, the visual system compensates for the
blind spots in each retina were the cells leave the eye by "filling
in" that area.
Functional Role Semantics
Whereas nomic covariationists focus upon a single
causal connection in fixing content, advocates of Functional Role Semantics
(Block 1986 and 1987, Field 1977 and 1978, Harman 1987), suggest that the
overall network of causal relations into which a state can enter fixes
its content. Often times causal roles are specified functionally/computationally.
Functional Role Semantics is also known as Conceptual Role Semantics, Procedural
Semantics, or Inferential Role Semantics. There are two versions
of this approach designated "wide" or "long-armed" and "narrow" or "short-armed".
Narrow functional role theorists limit the causal relations that determine
content to those occurring between mental states. Wide theorists allow
connections to the distal environment and even social contexts to delimit
the content of a state.
Functional role theories have several attractive
features. First, they do not need bifurcated accounts of representational
content since all states get their content in the same manner, via their
functional role. Hence, functional role theorists avoid explaining
the representation of high-level properties by defining them in terms of
low-level properties. Second, functional role theorists can easily
accommodate the observation that changes in beliefs can result in changes
in representational content, since changing beliefs will often change the
functional roles of states. Finally, functional role theorists can
capture the intuition that in trying to understand the representational
content of another cognizer's state, one is constrained by the heuristic
that the overall set of content ascriptions must "make sense," i.e., be
consistent with the supposition that overall, the cognizer's interactions
with the world are intelligent or rational.
Illustrative
Animation
Objection 1: Semantic Holism
Theorists typically raise three distinct but related
objections to all versions of Functional Role Semantics. First,
to identify particular states in disparate individuals as states having
the same representational content, Functional Role Semantics prima facie
requires that the states have identical functional roles. Fodor (1992,
1987) among others has termed this view "Holism," about content (also
"Semantic Holism"). For example, suppose that it appears that both
Bill and Bob believe that "Wallis' article is enlightening." That
is, they both point to the same article, proclaim that it is enlightening,
assign it in their class, etc.. However, the causal roles of their
respective states differ in a single respect; Bill believes "Wallis is
a pompous know-it-all," while Bob believes "Wallis is a precocious windbag."
Prima facie, Functional Role Semantics dictates that Bill's belief that
"Wallis' article is enlightening," has a different content than Bob's.
Similarly, people with disparate cognitive or perceptual abilities seem
to have disparate representational contents. Thus, the theory appears
to imply that the average human has a different concept of traffic light
than color blind humans.
Functional role semanticists generally adopt one
of two responses to the above objection. One response strategy
would prune the number and/or kind of causal or computational connections
necessary for belief/content identity, thereby allowing for belief/content
identity across individuals with somewhat different causal/computational
roles. In the above case, differences in beliefs that are peripheral
to the belief that "Wallis' article is enlightening" would not necessarily
constitute the basis for content non-identity.
While the just-rehearsed response has
intuitive appeal, critics (Fodor 1988) point to the potential difficulties
in distinguishing core (central) beliefs or other causal links from peripheral
ones in a manner that is not hopelessly unsystematic and ad hoc.
For instance, what causal connections (beliefs, desires, dispositions to
take action, etc..) constitute the core of one's belief that the colorless,
tasteless, odorless liquid before one is water. Need one know, to
take a case, of the existence of deuterium oxide (heavy water), make appropriate
inferences with regard to D2O, discriminate between D2O and
H2O, etc..
Including only analytic beliefs
and corresponding inferences in the set of core beliefs and inferences is one common suggestion for distinguishing
core from peripheral beliefs and inferences.
Analytic truths are conceptual truths, those things that are true solely
in virtue of the nature of the concept. Such a suggestion requires
a real distinction between analytic beliefs and non-analytic (synthetic)
beliefs. Many philosophers believe that Quine (1953/1970) has effectively
undermined the robustness of such a distinction. Similarly, Stich
(1983) argues that judgments of belief/content identity are not as intuitively
precise as advocates of the analytic distinction would seem to predict.
The second response to the prima facie difficulty
of intuitively identical content across differing functional roles claims
that while such beliefs are in fact non-content-identical, they are strongly
conten-similar. The key concept here is the notion that belief identity
is not a binary notion, but ranges from completely non-identical to completely
identical (Cummins 1989). The strength (or weakness) of this response
lies in its ability to accommodate the intuition that Bill's and Bob's
beliefs have the same content while simultaneously acknowledging the theoretical
constraint that differences in functional roles dictate differences in
content.
Critics of Functional Role Semantics (ex. Fodor,
1988) argue that a graded notion of content identity, in addition to being
counterintuitive, undermines the ability to formulate psychological generalizations
and subsume particular cases under those generalizations. Cognitive
Science, they claim, would be reduced to the unworkable notion that Bill's
and Bob's beliefs are, say, 97% content similar to the belief that Wallis'
article is enlightening and hence can be, say, 97% subsumed under generalizations
regarding the belief that Wallis' article is enlightening. Furthermore,
Fodor (1992 and 2001) claims colloquial notions of belief similarity like,
"His notion of mental representation is similar to mine," as well as their
theoretical counterparts presuppose a notion of belief identity that he
claims cannot be provided by holistic theories in any cases where beliefs
diverge.
Objection 2: Content Fixing, Error,
and Univocal Contents
A closely related objection to that of holism is
the argument that functional role theories have no non-arbitrary way either
of fixing content, distinguishing representational error from veridical
representation, and/or they result in non-univocal content ascriptions
or multiple content ascriptions applying simultaneously to a given state.
For example, suppose that there are two worlds: one, call it Earth1
in which there is no water, but in which D2O (or some chemically different,
but phenomenally similar substance) is plentiful; the other, call it Earth2,
where H2O is plentiful, but there is no D2O. Bob grows up on
Earth1,
where he forms beliefs, etc. about "water" as a result of interacting with D2O. Bill grows up on
Earth2, where he develops the exact same set
of beliefs, etc. about "water" as Bob, but based upon interacting with H2O. If one is a narrow functional role theorist (using only causal
connections "internal" to the cognizer), then the two men must have identical
belief content when thinking about "water". They either form beliefs,
make inferences, etc., using states that represent what we would describe
using the disjunction, "H2O or D2O", or their states represent both
H2O
and also D2O (as distinct entities) simultaneously. Most philosophers
consider the latter even less intuitively plausible than the former.
Long arm or two factor theories can distinguish
Bill's and Bob's beliefs since they include causal connections to environmental
objects and/or properties. However, suppose that Bob also has the
beliefs that "water is H2O," that "I live on Earth2," and
"Earth2 has only H2O on it's surface." Is Bob representing "water" as
D2O, but forming a false belief about the chemical structure of D2O?
Or, is he representing "water" as H2O and forming false beliefs about the
D2O he finds in his environment. Critics assert that Functional Role
Semantics lacks the resources to disambiguate such scenarios.
Objection 3: Representational
Structure
Finally, Fodor and LePore (1992), Fodor and
McLaughlin (19891), and Fodor and Plysyhyn (1988) raise the objection that
Functional Role Semantics seems to violate truths about the structure of
language and thought like compositionality and systemativity. Compositionality
is the theory that the meaning of a complex expression in a language results
from the meanings of its constitutive elements. Compositionality
plays an central role in many linguistic theories, since its supposition
for both language and thought provides a fairly straightforward explanation
of the human ability to grasp an enormous number of different thoughts
of varying complexity and their corresponding linguistic expressions.
For instance, because we understand the individual elements like "cup"
and "coffee", we understand the complex expressions "cup of coffee," "hot
coffee," "coffee gives me the shakes," etc.. One explains our understanding
by noting that the meaning of these complex sentences is built-up from
the meaning of their constitutive elements.
Fodor and others claim that the functional role
of a complex, non-idiomatic representation is not always a function of
the functional roles of its parts. As a result, functional role theories can represent "cup of
coffee" without having the concepts and associated inferences of "cup"
or "coffee". This alleged aspect of functional role representation
schemes would result in the possibility that one could represent and think
about "cup of coffee," but could not represent or think about "iced coffee,"
"hot coffee," etc.. Nor could one understand or evaluate the sentence,
"Coffee is the legal drug for which we exploit the people and natural resources
of many third world countries."
Block (forthcoming) has suggested that functional role semanticists can account for compositionality by adopting context-sensitive rules of use for words. That is, meanings of words can be understood as sets of inferences (functional roles) to to sentences containing the word and sets of inferences from sentences containing the word. Thus, "cup of coffee" does get its meaning from cup and is consistent with one understanding "iced coffee", etc.. Connectionists with commitments to functional roles and others (Smolensky 1991 and 1991a) have argued that connectionist systems can have constituents satisfying many of the criteria of compositionality without adopting traditional compositional representational frameworks.
Causal Theories and Cognitive Science
Cognitive Science proceeds in the absence of a resolution of the debate
over theories of representation. However, the adoption of either
Nomic Covariance Theories or Functional Role Semantics does shape the research
of individual cognitive scientists. Many important debates in Cognitive
Science have resulted from allegiance to one or the other causal theory.
For example, one aspect of the connectionist vs Turing compatible approaches
is that advocates of connectionist frameworks tend to adopt Functional
Role Semantics, while advocates of Turing-compatible frameworks tend to
adopt nomic covariation. Similarly, the debate over the nature of
mental imagery was drawn along covariance vs functional role lines.
It is widely supposed that the adoption of one or the other theory of mental
content by the majority of cognitive scientists will have a profound impact
on the field.